Compute 95 Confidence Interval. So for the GB the lower and upper bounds of the 95 confidence interval. Our best estimate of the entire customer populations intent to repurchase is between 69 and 91. Assuming you have the same order for all 10 instances the delivery takes 554 minutes on average with a standard deviation of 8499. The 95 confidence interval is 69 to 91.

Our 95 confidence interval for the month of July is 1012F 1035F. We then multiply this value by the standard error which is 12 and we get 2352. The tag ggplot2 has beed added as it is tengentially relevant. That is give the 25th and 975th percentile of your bootstrap replicates stored as bs_replicates. To calculate the confidence interval go through the following procedure. The computation of confidence intervals is completely based on mean and standard deviation of the given dataset.
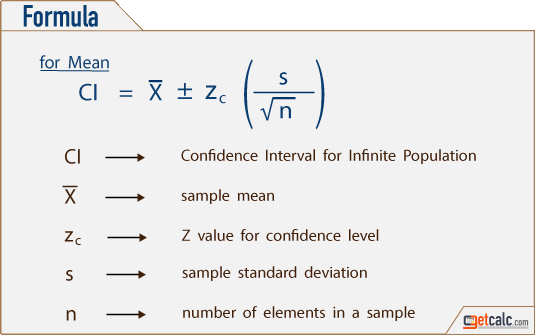
We get the values of z for the given confidence levels from statistical tables.
Confidence intervals address this concern as they provide a range of values which is likely to contain the population parameter of interest. Community Answer Work out the average standard deviation for your values and then the confidence Interval average and - 195 x standard deviation. Assuming you have the same order for all 10 instances the delivery takes 554 minutes on average with a standard deviation of 8499. The t value for 95 confidence with df 9 is t 2262. Because the sample size is small we must now use the confidence interval formula that involves t rather than Z. The 95 confidence interval defines a range of values that you can be 95 certain contains the population mean.